➔ Index of ⦁ Transmission Lines Theory ⦁
Transmission lines - Part V: Impedances
As we already introduced, impedance changes along a transmission line. Let's find a way to calculate it at every section of a transmission line!
Impedances
From the telegraphists' equation, two expressions for voltage and current along the transmission line have been found. Now, since Ohm's law is still valid, we can say that voltage and current are related by the following formula. $$Z(z)=\frac{V(z)}{I(z)}$$ But, as you see, we have a spatial dependency of the impedance on the line! Impedance is not constant (as usually is for DC or low frequency circuits), but changes with z variable: $$Z(z) = \frac{V_0^+e^{-jkt}+V_0^-e^{jkt}}{I_0^+e^{-jkt}+I_0^-e^{jkt}}$$ $$Z(z) = \frac{V_0^+(cos(kz)-jsin(kz))+V_0^-(cos(kz)+jsin(kz))}{I_0^+(cos(kz)-jsin(kz))+I_0^-(cos(kz)+jsin(kz))}$$ $$Z(z) = \frac{V_0^+(cos(kz)-jsin(kz))+V_0^-(cos(kz)+jsin(kz))}{Y_{\infty}V_0^+(cos(kz)-jsin(kz))-Y_{\infty}V_0^-(cos(kz)+jsin(kz))}$$ $$Z(z)= Z_{\infty}\frac{(V_0^++V_0^-)cos(kz)-(V_0^+-V_0^-)jsin(kz)}{(V_0^+-V_0^-)cos(kz)-(V_0^++V_0^-)jsin(kz)}$$ $$Z(z)= Z_{\infty}\frac{(V_0^++V_0^-)cos(kz)-Z_{\infty}(I_0^++I_0^-)jsin(kz)}{Z_{\infty}(I_0^++I_0^-)cos(kz)-(V_0^++V_0^-)jsin(kz)}$$ Dividing by cos(kz) and by (I0++I0-) we get a simpler formula: $$Z(z)= \frac{Z(0)-Z_{\infty}jtan(kz)}{1-Z(0)Y_{\infty}jtan(kz)}$$ Z(0) is the impedance at a known point of the transmission line (usually the load). Then, to go back to the generator we have to move backwards on the line by applying the length of the line stub to the tangent argument z.
Actually, using this formula during calculations by hand is not so comfortable so another tool can be used: the so-called Smith chart, explained in the next article.
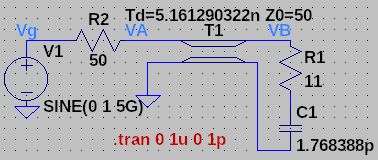
Example of transmission line with basic calculations
Navigate
Comments
Be polite and respectful in the comments section. In case of doubts, read this before posting.
Posted comments ⮧
Comment section still empty.
INDEX
INFO
STATISTICS
PREVIOUS ARTICLE
NEXT ARTICLE
CONTACTS
SHARE